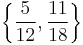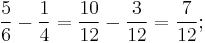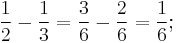Lowest common denominator
In mathematics, the lowest common denominator or least common denominator (abbreviated LCD) is the least common multiple of the denominators of a set of vulgar fractions. It is the smallest positive integer that is a multiple of the denominators.
The term is also used in popular culture with a different though related meaning.
Contents |
Examples
The LCD of
is 36 because the least common multiple of 12 and 18 is 36. Likewise the LCD of
is 12. Using the LCD (or any multiple of it, such as the product of the denominators) as a denominator enables addition, subtraction or comparison of fractions:
The lowest common denominator of two vulgar fractions can be found by calculating the least common multiple of their denominators.
Middle school instruction
Some K–1 math standards such as the latest revision of the NCTM math standards and reform mathematics textbooks created since the 1990s de-emphasize or omit coverage of the LCD entirely in favor of finding any common, but not necessarily the lowest common denominator, or by using less powerful methods such as fraction strips or "benchmark" fractions. The "cross-multiply" method of comparing fractions effectively creates a common denominator by multiplying both denominators together.
Algorithm finds lowest common denominator.
Lowest common denominator for 2/9 + 1/4 + 1/6:
Start with the 3 denominators in an upside-down division box. The algorithm uses similar division boxes going downward.
Start with 2 and see if it divides exactly into any of the three denominators. Then go to 3, then 4, then 5, and so on.
|_9_4_6_
2|_9_4_6_ 2 doesn't go into 9 exactly. 2 goes into 4 two times, and into 6 three times.
2|_9_2_3_ 2 goes into 2 one time.
3|_9_1_3_ 3 is the next divisor. 3 goes into 9 three times, and into 3 one time.
3|_3_1_1_ 3 goes into 3 one time.
|_1_1_1_
The process is to keep dividing the denominators until they reduce to 1. Then ignore the 1's and use the column of divisors as factors which produce the L.C.D.
2 x 2 x 3 x 3 = 36 = L.C.D.
Non-mathematical usage
'Lowest common denominator' is often used as a figure of speech meaning the most basic, least sophisticated level of taste, sensibility, or opinion among a group of people.
This popular sense of the term is not faithful to mathematical usage. Because the figure of speech conveys the sense of degrading by eliminating anything not common to all (but not degrading further), a better mathematical metaphor for the colloquial usage's connotation is 'greatest common divisor.' However, it seems that the word greatest strikes non-mathematicians' intuition as suggesting the lofty, rather than the base.
See also
- Greatest common divisor
- Partial fraction expansion — reverses the process of adding fractions into uncommon denominators.
- Anomalous cancellation